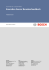
( )2 ∫ c. ∫ − ( ) ∫ b. ∫ − ∫

3 Integral calculus
Englische Aufgaben zum Kapitel 3 Integralrechnung
3.1 Which of the functions F are antiderivatives of the given polynomial, trigonometric and exponential
functions f, respectively?
a. f (x ) = 3x 3
A F (x ) = 9x 2
C F (x ) = 3x 4 − 7
D F (x ) = 43 x 4 + 12
C F (x ) = − 31 cos(3x ) − 3
D F (x ) = cos(3x )
C F (x ) = 31 e3x
D F (x ) =
B F (x ) = 0.75x 4
b. f (x ) = sin(3x )
( )
A F (x ) = − cos 23 x 2
B F (x ) =
( )
− cos 3 x 2
2
c. f (x ) = e 3x
A F (x ) = e
3 x2
2
B F (x ) = 3e 3x
e 3x +5
3
[antiderivative … Stammfunktion]
3.2 Match each function f to the graph of an appropriate primitive integral F.
a.
b.
c.
A
B
d.
C
D
[primitive integral … Stammfunktion]
3.3 Draw the graph of an antiderivative F of the function f satisfying the condition F (a) = b .
a. f (x ) = 3x 2 , a = 0, b = -2
b. f (x ) = cos(x ) , a =
π
2
,b=5
c. f (x ) = 8x + 8 , a = −4 , b = 0
3.4 Compute the indefinite integral.
a.
∫ 3x
2
− 5x + 3x dx
b.
∫ 3xy
2
− 5 yx 3 + y dy
∫ 5ab
5
c.
− a + sin(ab ) db
[indefinite integral … unbestimmtes Integral]
3.5 Compute the indefinite integral. Decide whether you have to use one of the following techniques:
integration by parts, integration by substitution or integration by partial fractions.
a.
∫
5
( x + 2 ) ⋅ ( x − 1)
b.
∫
x 2 + 4 x − 12
2x + 1
dx
dx
c.
∫
d.
∫e
dx
e.
∫ x ⋅ (4x
⋅ (2x − 1) dx
f.
∫
2x + 3
x 2 + 3x − 8
x
2
+2
)
25
dx
4 x 2 + 3x − 1
dx
x2
[integration by parts … partielle Integration; integration by substitution … Integration durch Substitution;
integration by partial fractions … Integration mit Partialbruchzerlegung]
© Österreichischer Bundesverlag Schulbuch GmbH & Co. KG, Wien 2014 | www.oebv.at | Mathematik
Alle Rechte vorbehalten. Von dieser Druckvorlage ist die Vervielfältigung für den eigenen Unterrichtsgebrauch gestattet.
Autorin: Bettina Ponleitner
3 Integral calculus
Englische Aufgaben zum Kapitel 3 Integralrechnung
3.6 Write down a formula using definite integrals for computing the coloured area and compute the area.
a.
b.
c.
f( x ) = −x 2 + 4
f ( x ) = − 21 x 2 + 4 , g( x ) = x 2 − 2
f ( x ) = − 21 x 3 + 3x − 1 , g( x ) = e x − 2
[definite integral … bestimmtes Integral; area … Fläche]
3.7 Plot the function f: R¥R, f(x) = x4 – 5x² + 4. Decide whether the following statements are true or false.
1
a.
∫
2
∫
f ( x ) dx = f ( x ) dx
−2
1
2
b. The area enclosed by f and the x-axis is A =
∫ f(x)dx .
−2
c.
d.
1
1
−1
2
0
∫ f(x) dx = 2 ⋅ ∫ f(x) dx
∫ f(x ) dx < 0
0
−1
f. The area enclosed by f and the x-axis in [-2; 0] is A =
∫
−2
0
∫
f ( x ) dx + f ( x ) dx .
−1
[to enclose … einschließen; x-axis … x-Achse]
3.8 The acceleration of a car at t seconds is a( t ) = −0.0003t 2 + 0.08t + 2 for t in [0; 14].
a. Find a function v for t in [0; 14] describing the cars’ velocity at time t in m/s, if the cars’ velocity at
t = 0 is zero, and compute the velocity at t = 7.
b. Find a function s which for all t in [0; 14] gives the covered distance in [0; t] in meters.
c. Compute the distance the car has covered after 12 sec.
d. After 14 seconds the car slows down again. The deceleration due to braking is 6.5m / s 2 . Find a
function v b which gives the velocity of the car at time t during the slowing-down process. Compute the
time until the car stops as well as the covered distance.
[acceleration … Beschleunigung; covered distance … zurückgelegte Strecke; to slow down … bremsen;
deceleration due to braking … Bremsverzögerung; slowing-down process … Bremsvorgang]
3.9 Compute the mean value of f ( x ) = 3x 3 − 15x in [0; 4].
3.10 A cup of tea is left to cool down. The process of cooling down is described by the function T with
T ( t ) = 63 ⋅ e −0.15⋅ t + 25 , where t is the time in minutes and T(t) the temperature of the tea in °C.
a. Compute the temperature of T at t = 0 and at t = 10.
b. How long does it take until the temperature has dropped to approximately 30°C?
c. Compute the average temperature of the tea in the first 8 minutes.
[to leave to cool down … abkühlen lassen; to drop … absinken]
© Österreichischer Bundesverlag Schulbuch GmbH & Co. KG, Wien 2014 | www.oebv.at | Mathematik
Alle Rechte vorbehalten. Von dieser Druckvorlage ist die Vervielfältigung für den eigenen Unterrichtsgebrauch gestattet.
Autorin: Bettina Ponleitner
3 Integral calculus
Englische Aufgaben zum Kapitel 3 Integralrechnung
3.11 We know several function values of a continuous function f: f(0) = 0; f(3) = 46.5; f(6) = 228.
a. Compute the integral approximately using (1) the midpoint rule, (2) the trapezoidal rule and (3)
Simpson’s rule.
b. Assume, the equation of f is given by f ( x ) = 21 x 3 + 3x 2 + 2x . Compute the exact integral of f and the
absolute error between each approximation and the true value of the integral.
[midpoint rule (or rectangle rule) … Rechtecksregel; trapezoidal rule … Trapezregel; Simpson’s rule1 … Kepler’sche Fassregel;
remark … Bemerkung]
3.12 Use the composite Simpson’s rule and compute the integral
∫
6
0
x 3 ⋅ e − x dx for n = 3.
3.13 The internal shape of a ceramic vase is given by the function f ( x ) = 0.3x 2 for x ∈ [0; 10] rotating about
the y-axis. The exterior of the vase is given by a straight line through (8 | 0 ) and (10.5 | 30 ) , rotating
about the y-axis for y ∈ [− 5; 30] (unit: centimeter).
a. Draw the profile of the vase.
b. Compute the volume of the vase if it is filled up to 5 cm below the brim.
c. Calculate the mass of the empty vase, if the density of ceramic is ρ = 3.23g / cm3 .
[internal shape … Innenform; ceramic vase … Keramik-Vase; exterior … äußere Form; brim … Rand; density … Dichte]
1
Remark: In German, the formula
Fassregel and the formula
b
b
∫ f(x) dx ≈
a
∫ f(x) dx ≈
a
b −a
6n
1
6
[
⋅ (b − a) f (a) + 4 ⋅ f
(a+2b ) + f(b)] is called Kepler’sche
[f(a) + 4 ⋅ f(a1 ) + 2f(a2 ) + 2 + 4f(a2n− 1 ) + f(b)] is called
zusammengesetzte Fassregel or Simpson-Regel (see Mathematik HTL 3, chapter 3.4, for details).
However, in English the formulas are called Simpson’s rule and composite Simpson’s rule, respectively.
© Österreichischer Bundesverlag Schulbuch GmbH & Co. KG, Wien 2014 | www.oebv.at | Mathematik
Alle Rechte vorbehalten. Von dieser Druckvorlage ist die Vervielfältigung für den eigenen Unterrichtsgebrauch gestattet.
Autorin: Bettina Ponleitner
3 Integral calculus: solutions
Lösungen zu: Englische Aufgaben zum Kapitel 3 Integralrechnung
3.1 a. B , D
b. C
3.2 a. B
3.3
c. C , D
b. D
c. A
d. C
b. F (x ) = sin( x ) + 5
a. F (x ) = x 3 − 2
c. F (x ) = 4 x 2 + 8x
(
2
)
3.4 a. x 3 − 5 x2 + 3 ln x + c
b. xy 3 − 21 ⋅ 5x 3 y 2 − y 2 + c
3.5 a. integration by partial fractions:
∫
5
( x + 2 ) ⋅ ( x − 1)
b. integration by partial fractions:
∫
x 2 + 4 x − 12
2x + 1
c.
5b 6 a
6
dx = 53 ⋅ (ln x − 1 − ln x + 2 ) + c
dx = 58 ln x − 2 +
c. integration by substitution ( x 2 + 3x − 8 = u ):
∫
2x + 3
x 2 + 3x − 8
11
ln x
8
+6 +c
dx = ln x 2 + 3x − 8 + c
d. integration by parts: e x ⋅ (2x − 1) dx = (2x − 3) ⋅ e x + c
∫
∫ (
)
e. integration by substitution ( 4 x 2 + 2 = u ): x ⋅ 4 x 2 + 2 dx =
f. neither of the above methods:
∫
4 x 2 + 3x − 1
dx
x2
∫
= 4 + 3x −
1
x2
(4 x
2
+2
208
)
26
+c
dx = 4 x + 3 ln x + x1 + c
© Österreichischer Bundesverlag Schulbuch GmbH & Co. KG, Wien 2014 | www.oebv.at | Mathematik
Alle Rechte vorbehalten. Von dieser Druckvorlage ist die Vervielfältigung für den eigenen Unterrichtsgebrauch gestattet.
Autorin: Bettina Ponleitner
− ba − cos(aab ) + c
3 Integral calculus: solutions
Lösungen zu: Englische Aufgaben zum Kapitel 3 Integralrechnung
3.6 a. A =
∫
−2
∫
2
f ( x )dx + 2 ⋅ f ( x )dx =13
−3
0
2
∫ (f(x) − g(x))dx =16
c. A = ∫ (g( x ) − f ( x ) )dx + ∫ (f ( x ) − g( x ) ) ≈3.52
b. A = 2 ⋅
0
0
1
−2
0
3.7 a. true
−1
b. false; the correct answer is: A = 2 ⋅
∫
1
∫
f ( x )dx + f ( x )dx .
−2
−1
c. true
2
∫
d. false; the correct answer is: f ( x ) dx > 0 .
0
f. true
3.8 a. v( t ) = −0.000075 ⋅ t 4 + 0.04 t 2 + 2t ; v( 7 ) ≈ 15.78m / s ≈ 56.81km / h .
b. s( t ) = −0.000015 ⋅ t 5 + 0.013333 ⋅ t 3 + t 2
c. s( 12) = 163.3m
d. v b ( t ) = v( 14 ) − 6.5t = 32.9588 − 6.5t . The car stops after about 5.07 seconds. The distance covered is
s b (5.07 ) =
5.07
∫ 32.9588 − 6.5t dt = 83.56 m .
0
3.9 mean value of f in [0; 4]: 18
3.10 a. T(0) = 88°C; T(10) = 39°C
b. t ≈ 16.9s
c. average temperature: t av ≈ 61.69°C
6
6
0
6
0
∫
∫ f(t )dt ≈ 6 ⋅
(3) Simpson’s rule: ∫ f ( t )dt ≈ ⋅ 6 ⋅ [f (0) + 4 ⋅ f (3) + f (6 )] = 414
b. exact integral: ∫ ( x + 3x + 2x )dx = 414 ;
3.11 a. (1) midpoint rule: f ( t )dt ≈ 6 ⋅ f (3) = 279 , (2) trapezoidal rule:
1
6
0
6
1
0 2
f ( 0 ) + f (6 )
2
3
2
error by computation with midpoint rule: 414 – 279 = 135; error by
computation with trapezoidal rule: 414 – 684 = -270; error by
computation with Simpson’s rule: 414 – 414 = 0.
3.12 For n = 3 we have 6
subintervals.
6
∫ f(t )dt ≈
0
6 −0
18
⋅ [f (0) + 4 f ( 1) + 2f ( 2) + 4 f (3) + 2f ( 4 ) + 4 f (5) + f (6 )] ≈ 5.09
[subinterval … Teilintervall]
3.13 b. Vint erior = π ⋅
25 y
0 0.3
∫
dy ≈ 3272.5cm3
∫ (
)
30 y
2
 30 y

c. g : y = 12x − 96 ; Vvase = π ⋅ 
+ 8 dy −
dy  ≈ 4354.6cm3
12
0
.
3
0
 −5

mass of the empty vase: m = ρ ⋅ V = 14065.5g ≈ 14kg
∫
© Österreichischer Bundesverlag Schulbuch GmbH & Co. KG, Wien 2014 | www.oebv.at | Mathematik
Alle Rechte vorbehalten. Von dieser Druckvorlage ist die Vervielfältigung für den eigenen Unterrichtsgebrauch gestattet.
Autorin: Bettina Ponleitner
a.
= 684